Introduction to the Related Rates Calculator
The related rates solver is a powerful online tool designed to assist students and enthusiasts in solving related rates problems in calculus. Whether you're dealing with scenarios involving cones, circles, spheres, cylinders, rectangles, or even cubes, this calculator simplifies the process of calculating how different variables change in relation to each other.
Understanding Related Rates Problems
Related rates problems involve analyzing the rate of change of one quantity concerning the rate of change of another related quantity. These problems often deal with changing dimensions or variables in different shapes, such as cones, circles, and spheres. The related rate calculator is your ally in tackling these problems efficiently.
The formula used by the related rates square calculator
Here's the formula used by related rates calculator calculus:
\[ \frac{dA}{dt} = 2\pi \cdot r \cdot \frac{dr}{dt} \]
How the Related Rates Calculator with steps Works
The related rate calculator employs fundamental calculus principles to handle related rates problems. It calculates derivatives to find rates of change and provides a step-by-step analysis of the problem. Let's look at an example using a circle:
Example: Calculating the Rate of Change of a Cone's Area

Imagine a cone with a radius of 5 units. As the radius changes, the area of the cone also changes. The Related Rates Calculator can determine how quickly the area is changing concerning the rate of change of the radius.
Using the Related Rates Calculator with Steps
- Input the initial values of the variables (in this case, the radius of the circle).
- Specify the rate of change of the given variable (how fast the radius is changing, for instance).
- The calculator will compute the derivative of the formula for the area of a circle, which is A = π * r^2.
- It will then substitute the values and rates of change into the derivative formula.
- The result will provide the rate of change of the circle's area concerning the rate of change of its radius.
Benefits of the related rates solver
Efficiency: Solving related rates problems manually can be time-consuming and complex. The calculator streamlines the process, saving you time and effort.
Comprehensive Steps: The calculator breaks down each step of the calculation, making it easier to understand the solution.
Multiple Scenarios: Whether it's cones, circles, spheres, cylinders, rectangles, or cubes, the calculator handles various shapes and scenarios.
Accurate Results: The calculator employs precise calculations, ensuring accurate results for your related rates problems.
Utilizing the Related Rates Calculator with Solution
- Input the given values and rates of change.
- Specify the variables and their relations in the problem.
- The calculator will calculate derivatives and provide step-by-step solutions.
- Obtain the solution, which showcases how the variables change in relation to each other.
Solving Real-world Scenarios
The Related Rates Calculator is not only a mathematical tool but also a problem-solving tool for real-world situations. Whether you're calculating the rate at which an ice cube melts or how the dimensions of a changing cone evolve, this calculator is your go-to resource.
Frequently Asked Questions
Can the related rate calculator handle different shapes and scenarios?
Absolutely. This calculator is versatile and can handle various scenarios involving shapes like cones, circles, spheres, cylinders, rectangles, and even cubes. Whether you're dealing with melting ice cubes or changing the dimensions of different objects, the calculator can assist you.
Is the Related Rates Finder suitable for beginners in calculus?
Yes, the calculator is designed to be user-friendly and accessible to beginners. It provides detailed steps and explanations, making it easier to understand the solution process for related rates problems.
How accurate are the results provided by the Related Rates Calculator?
The calculator employs precise mathematical calculations and formulas, ensuring accurate results for your related rates problems. However, it's important to input the correct initial values and rates of change for accurate solutions.
How do I interpret the results provided by the related rates solver?
The calculator will provide you with numerical values that represent the rates of change of different variables. These values indicate how quickly one variable is changing concerning another variable. It's important to consider the context of the problem to interpret the results accurately.




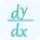












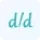
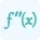
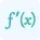
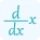






 Google Play
Google Play

0 Comment