Introduction to Linear Approximation Calculator
Linear approximation calculator uses linear function to calculate a general function. You can calculate the linear approximations of parametric, polar, or explicit curves at a given point. Using manual calculations can take a lot of time so linearization calculators help us evaluate the derivative to find the slope of entered points.
Formula used by Linearization Calculator
The linearization calculator uses a linear approximation formula to find the function. This will enable you to evaluate the derivative of a function to find the slope of a curve. Since a linear approximation calculator uses the same linear approximation formula, you just need to enter your value and the tool will automatically provide accurate results.
The linear approximation formula used by this tangent line approximation calculator is:
$ y \;=\; f(a) \;+\; f'(a)(x-a) $
You can use this linear approximation formula to calculate manually or use our tool to calculate digitally as well.
How to find a Linear Approximation Calculator?
There are 2 ways through which you can find a local linearization calculator.
- Search on Google by using the keyword "linear approximation calculator". Google will show you a lot of results and now you have to pick wisely. Pick a result from Google which is easy to understand and use. Which accurately finds the slope of given points.
- Search the differentiation calculator and find your approximate value calculator from here. There are a lot of other tools like partial differential calculator on this website as well. This website will help you understand the different differentiation calculations with its online and accurate calculators.
Why to use Linearization Calculator?
Differential calculus is one of the most technical and difficult concepts within mathematics. Approximate linearization is also very tricky. If you have good concepts and practice, you can deal with it easily. Otherwise, you need some external help to understand and calculate.
Linear approximation calculator provides step by step results which can help you understand this concept more effectively. You can use a lot of different examples to calculate and understand. This practice will be very crucial for your overall learning of this concept. That is why it is very beneficial for you to use a tangent line approximation calculator.
Benefits of using Linear Approximation Calculator
It is always beneficial and smart to use an approximate value calculator for learning and practice. Some of the major benefits of this calculator are:
- It saves your time you spend on doing manual calculations.
- This differential approximation calculator is simple and easy to use.
- You can do practice to consolidate your linear approximation concepts.
- It provides accurate and step by step results.
- It provides plot and possible intermediate steps of linear approximation.
- You don't need any fee or subscription to use this linearization calculator.
How to use Linear Approximation Calculator step by step?
It is very simple and easy to use this linear approximation formula calculator. Just follow below steps to calculate linear approximation step by step:
- Load an example if you don't have one to calculate.
- Enter a function of which you want to find linear approximation.
- Enter a point (value) to find the value function at the given point.
- Verify whether your values are correct.
- Click on the "Calculate" button.
Immediately after clicking on the button, the tangent line approximation calculator will show you accurate step by step results along with plot and possible intermediate steps.




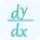












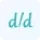
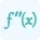
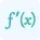



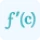



 Google Play
Google Play

0 Comment